I've become a bit obsessed with ratio tables this year. After the successes of teaching percentages with them last year, I knew the next topic I needed to try them with was compound measures. My year 9's first encounter with ratio tables was basic proportion, things like best buys, recipes and exchange rates, so students were confident using ratio tables before looking at compound measures. The series of lessons I planned on speed and then density went down really well with my students using a ratio table approach. The next topic happened to be linear graphs, and I soon realised I could use ratio tables to find the gradient in the same way, and explain it to students in the same way as compound measures. The need for compound measures to have a standardised unit of 1 to be able to compare really helped students see the multiplicative relationship between all three of these topics. Hence my realisation that the gradient was a compound measure - an epiphany which really changed how I approach teaching gradient.
Below is some of what I did and what I learned:
1. Speed
I gave them a prompt to start off, one that I'd seen in my Mastery training, couldn't find so remade my own version (apologies to whoever originally did it, if anyone knows who it was let me know so I can credit them). The question was who is fastest given the times and distances:
I asked students to justify their answers. Some students had already encountered the speed distance time formula, so went straight in with that. I asked them show me another way, rather than just calculating the speed, which really stumped them. Students with no concept of speed could readily compare the Tim and Adam with the same distance (Adam runs the same distance in less time so faster than Tim) and could readily compare Sam and Tim with the same time (Sam runs further in same time so faster than Tim). The issue was comparing the two with nothing the same (Sam and Adam) and students were starting to understand the compound nature of speed and the need for one unit to be the same to compare.
As a class we then discussed some different methods. All of the different methods showed how Sam is fastest. I love opportunities to embrace different methods, and this provided a great opportunity. There were lots of "so how else could we show it?" questions posed. I purposefully avoided those students who had calculated the mean at first. I picked students who had multiplied up and used lowest common multiple to create the same distances or same times so then they could compare who either ran furthest if times were the same or who took the least amount of time if they ran the same distances (a bit like when doing best buy questions, using the lowest common multiples to create either the same amount or the same price to then compare the prices or amounts).
The ratio tables really helped support showing these different methods. Students were able to justify who was fastest and who was slowest as they'd made either the distance the same or the time the same. Students were keen to tell me the speed, but some already had misconceptions using the formula triangles to divide time by distance instead. So I encouraged the ratio tables to support them in finding the speed. If they make the time 1 second for each person, we then have meters per second and that makes them comparable.
Then it completely threw some students when I suggested we make them all run 1m and compare the time instead. "That isn't speed though miss!". To which I replied "I wasn't asking you to find their speeds, I was asking you to find the fastest, so I didn't need to use the speed as all of these methods have helped show who is fastest". This then lead to a discussion that most of them had picked the most common method (method 4) and the method that we've all come to understand as speed as a universal way to communicate how fast things/people move, that we make the time a standardised 1 hour or 1 second and adjust the distance, the units are miles per hour (miles in one hour) kilometres per hour (km in one hour) meters per second etc. We make the time one unit so we can compare the different distances travelled in the standardised amount of time.
On reflection, I would change the initial problem to be "order them from slowest to fastest" rather than just who is fastest, and would suggest from the start I would like at least 2 different ways of justifying your answers. This is the first time I've introduced speed in this way and the impact of choosing this task as an introduction I felt really improved student understanding of what speed is, how it is compound in nature, the need to standardise to compare. Those with prior knowledge of the formula appreciated where the formula came from (as some of them remembered the formula incorrectly previously).
We then looked in isolation at finding speeds when given distance time, I tried to vary the questions. The ratio tables weren't given, but revealed one by one if students needed the guidance. I purposefully chose some more awkward times for students to work with too, although avoided really tricky times:
Upon reflection, here I would likely try to add a few that were more difficult times, such as an hour ten minutes, 54 minutes. Times which maybe would benefit from using a longer ratio table, to break down the time first to then multiply up to one hour (more on this below, keep reading!).
We then looked at finding distances given the speed and time. Students initially struggle with putting the speed in the table and need a bit of guidance, if it is 5m/s then how far do they go in what time? Is a good nudge, as well as emphasising the units "it is 5 METERS per 1 SECOND".
We then looked at finding the time given speed and distance.
These questions particularly lend themselves better to the breaking it down to build it up method (again, keep reading!) but also thinking about the connection across the table rather than down the table:
We then did a mixture including tricky units with the time (20 minutes and other fractions of hours). I've seen my wonderful colleague convert hours straight to minutes to help support students with this barrier- something I'm keen to do in the future having seen how some students really struggle with time being base 60 (rather than my approach of "what fraction of an hour is it?"). Whilst trying to highlight the most efficient ways of doing the questions (in a 2 by 2 ratio table) I always showed them alternative ways which would maybe be more suitable to non-calculator questions, where you break the units down to build them up to what you need. Some of the trickier questions students found easier if you broke the unit you wanted down to one and then built it up to the correct number (essentially the unitary method they'd previously come across, see example method on the right below):
I think it is beneficial for students to see a variety of methods so they can see how flexibly the ratio tables can be used. Some methods lend themselves better to calculators and the breaking down to 1 to build up can eventually be moved into one step as students become more confident (division is the denominator of a fraction, multiplication is the numerator). Eventually students can move away from the ratio tables as their understanding of speed develops, but they always have them to fall back on if needed. We also did some more complex questions like having two parts of a journey and finding the average speed, some I've collected from exam papers across the years, and converting from m/s to km/h and km/h to m/s (again, loving Dr Austin's worksheet on converting speeds).
2. Density
I linked this to speed in the sense of we need to 'standardise' the volume to be 1cm cubed of the substance to see what the mass was. This came about from discussing with my Science colleagues how they teach density, and they provide 1cm cubed of different metals for students to weigh, and students discover that some are heavier than others despite being the same volume, as they have more densely packed particles. I discussed this with students to activate their prior knowledge and link what they've seen in Science to what we would be doing in Maths.
Students actually found density easier than speed, but maybe this was because they'd already practiced using the ratio tables with speed problems, they'd already got to grips with the compound nature of speed which transferred well to density or that they didn't have the issue of time being base 60 to deal with.
I adapted the great Don Steward's density resources to make the numbers slightly easier as an introduction and then used his own questions after:
I then used Helen Konstantine's (aka MathsHKO) grid density questions available here (with lots of other great compound measure resources) as the numbers were nicer to get them started and had a lot of variety. I then used Dave Taylor's Increasingly Difficult Problems available here. I love these intelligent practice questions - you can tell they have been carefully considered and have years of teaching experience behind them, perfect resources ready to use in the classroom to help with the planning burden!
3. The Gradient
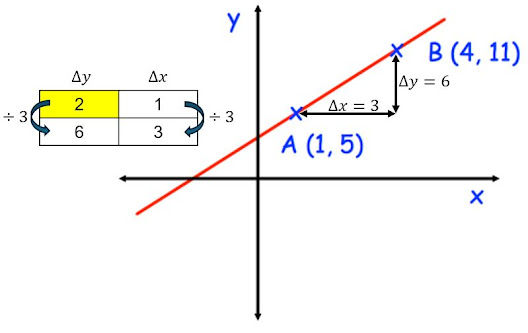
Now this is the one I stumbled across accidentally and coincidentally LOVED. It fit in really well as it was the topic after proportion and compound measures, the students were confident using ratio tables. Because of how I'd set out the speed and density ratio tables, and for consistency, I made the conscious decision to put change in y on the left and change in x on the right which seems counter intuitive. However, I think it worked as the density had 1 in the top right box and I felt the consistency would help. Maybe I should have also done this with speed too?
We looked at physical graphs first, and they could remember from previous years that the gradient was the steepness of the line. So I said well which is steeper and how do you know? They could tell me as you go across the steeper line goes further up compared to the other line. So I suggested it might be helpful to 'standardise' one of the directions to be 1 unit, just like in speed we use 1 hour or 1 second, just like in density we use 1cm cubed or 1m cubed. With a standardised change in x, we can then have a comparison for the change in y. Just like with the initial speed problem, we could either make time the same or distance the same to compare but the Mathematical consensus is to find 1 hour or 1 second and to force the time to be 1, and with density we compare 1 cubic centimetre or metre, the Mathematical consensus for steepness is to make standardise the change in x to be 1, which is what we know as the gradient.
I then picked a couple of different 'triangles' on the same lines to look at the change in y and the change in x in ratio tables, to show the gradient is the same in each, so when we 'standardise' to make the change in x one, we get the same change in y. They can physically see this in the graphs when they have the diagrams. We practiced a couple like this and I strongly recommended they use the ratio tables, and then use the one across is how many up or down method to check their answer (I love Dr Austin's finding gradients worksheet: Gradients from Lines).
We then moved on to gradient between two points with a sketch given (thanks to Corbett Maths for the idea of how to gently progress the types of questions and I borrowed plenty for my lessons: Corbett Gradient). I really emphasised the change in x and change in y in the ratio table as the difference in the x coordinates and the difference in the y coordinates. They practiced quite a few like this before removing the diagrams and going straight for gradient between two coordinates.
Some students were able to deduce a 'formula' of sorts, as we always ended up doing the change in y divided by the change in x, so they moved away from the tables which is great! The ratio tables are a scaffold which allow students to see the structure, so when they get to a point they realise to 'standardise' the change in x to always be 1 they end up dividing by the change in x, they understand fully what the gradient is and how to calculate it. Otherwise, from experience if you just tell them the formula they forget it or get it the wrong way round. They also were able to appreciate where the y2 - y1 / x2 - x1 formula comes from too (some of them having encountered in previously as a formula with no knowledge of why it works), knowing where a formula comes from or better supporting students to derive it themselves ensures they know how to use the formula and that they remember it correctly.
My next topic is similarity, which also includes trigonometry - I'm now really looking forward to unleashing ratio tables in those topics too. Watch this space!